Acoustic Wave Propagation

Chapter 2: Acoustic Wave Propagation

 

• Basics

• Sound waves require a medium to propagate. As a sound wave propagates, the particles of the medium are displaced from the equilibrium positions. In addition, the internal elastic force of stiffness, the restoring force, and the inertia of the medium result in oscillatory vibrations.

 

• If the displacement of the particle is along the line of the propagation direction, such a wave is called longitudinal (compressional) wave. In other words, the medium expands or contracts in the same direction as the propagation direction. Most sound waves in fluids are longitudinal in character.
  

• If the displacement is perpendicular to the propagation direction, the wave is called shear (transverse). In other words, motion of a particle is transverse to the propagation direction (e.g., bending of a material). Shear waves exist in solids and very viscous liquids. There is no change in volume or density of the material in a shear wave mode.

• Displacement and Strain

• Suppose the plane zo is displaced to a plane z’=zo+w, w is called displacement. At some other point in the material (zo+L), the displacement w changes to w+dw. We are interested in the displacement variation (dw) as a function of z.
• Compressional strain : using first order Taylor expansion, we have

 

 ,
 (compressional).

The parameter S is defined as strain, it represents the fractional extension of the material. Longitudinal motion changes the cube volume by dw*A, where A is the area of the cross section. Therefore, the relative change in volume is dw/L = S since the total volume of A*L.

• Shear strain : similarly, we can define a shear strain (wave propagates in z and particles displace in y) as

 

 (shear) .
Note that there is no change in area (volume) and density as shear motion distorts it.

 

• Stress

• Stress is defined as the force per unit area applied to the object.

 

 

• Note that longitudinal stress is positive in the +z direction and negative in the -z direction, it is also the negative of pressure. The net difference between the external stresses applied to each side of the object is . Therefore, the net force applied to move a unit volume of the material relative to its center is .

 

• Hooke’s Law and Elasticity

• Assuming a 1D system and small stresses, Hooke’s law states that the stress is linearly proportional to the strain

 

T=cS,

where c is the elastic constant of the material.

• In practice, waves propagate in three dimensions. Therefore, stress, strain and elastic constants become tensors. Conventions for tensor notations and corresponding reduced notations are listed below (x,y and z denote three space dimensions).

 

Tensor notation	Reduced notation
xx	1
yy	2
zz	3
yz=zy	4
zx=xz	5
xy=yx	6

• The stress  and the strain  are second ranked tensors, the elastic constant   is a fourth ranked tensor. Note that both the stress and strain tensors are symmetric. Given the above notation, Hooke’s law becomes

 

.

• Most biological tissues are often modeled as isotropic materials. In this case, the above matrix notation reduces to

 

.
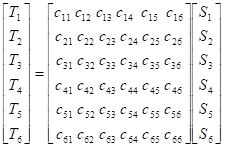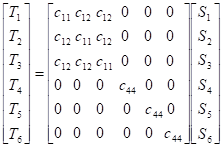In addition,
.

The above equation holds because when a material is compressed in one direction, it tends to expand in a perpendicular direction for an isotropic material and small displacements. Thus, only two independent elastic constants are needed for an isotropic medium. These two parameters are also known as the Lamé constants  and . Note that  is the ratio of the longitudinal stress in the z direction to the longitudinal strain in the y direction. m is also called the shear modulus (or modulus of rigidity).

• Young’s (elastic) modulus:

 

,

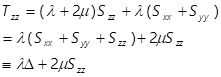where D is defined as dilation, representing the fractional change in volume.
Since shear stresses are not supported in fluids, by setting Txx and Tyy to be zeros, we can obtain the Young’s modulus (E):

.

• Bulk modulus: it is the reciprocal of compressibility, defined as the ratio of the pressure to the negative of the normalized change in volume. Therefore,

,
where
.

It is then straightforward to see that

• Poisson ratio: it is the negative of the ratio of the transverse compression to the longitudinal compression. Putting Txx = Tyy =0, we have

 

 

• Ultrasonic elasticity imaging

• The human sense of touch has been one of the most important medical diagnostic techniques. It is also a primary screening method for many pathologies, including breast cancer. However, palpation has been limited to lesions relatively close to skin surface and has been a very subjective technique. It is therefore of great importance to be able to detect deep lying, low contrast lesions, such as scarred renal tissue, in a quantitative fashion.

 

• The contrast of elasticity imaging is based on tissue elastic properties (e.g., Young’s modulus or shear modulus). The following figure shows variations of shear modulus for various body tissues. The shear modulus varies over a wider range than Bulk modulus, which is strongly related to conventional B-mode imaging. Therefore, elasticity imaging has the potential to dramatically improve the ability of tissue differentiation over current imaging methods. In other words, low contrast lesions (i.e., lesions with similar acoustic impedance as the surrounding tissue) which are not detectable in B-mode may be detectable using elasticity imaging.

 

• The general steps in elasticity imaging include
• Static or dynamic deformations using externally applied force.
• Measurements of internal tissue motion using conventional imaging techniques.
• Estimation of elastic properties of tissues.

 

 

 

 

• In order to simplify the Young’s modulus reconstruction, it is often assumed that soft tissue is incompressible (i.e., the total volume does not change due to deformation) and does not support shear stresses (i.e., ). Therefore,  and the elastic properties can be described by a single parameter.

• Challenges that need to be overcome before a clinical elasticity imaging system can be realized include:
• Deformation is hard to control in clinical situations.
• It is a more complicated three-dimensional problem in practice.
• Lateral displacements are more difficult to measure using phase sensitive speckle tracking techniques.
• Speckle decorrelation due to structure deformation.
• Computational complexity.

 

• Wave Equations

 

• Derivation of the ultrasonic wave equation can be understood by using the analogy between simple electrical and mechanical resonance circuits.

 

 

 

 

 

• The motion equation for the electrical system is (based on Kirchhoff’s voltage law)

,
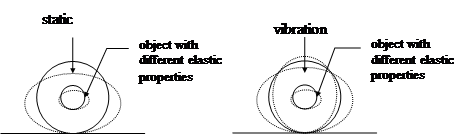
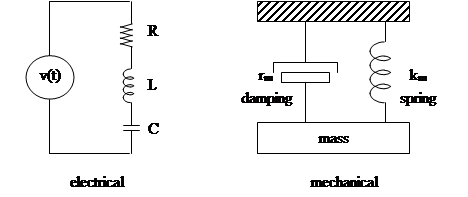
the motion equation for the mechanical system is (based on Newton’s second law)
,
where the analogies are

Electrical		Mechanical
q	charge	w	displacement
i=dq/dt	current	U=dw/dt	particle velocity
V	voltage	f	force (stress, pressure)
L	inductance	m	mass
1/C	1/capacitance	km	stiffness
R	resistance	rm	damping

• For a continuous medium, the mechanical motion equation can be modified as follows. Assuming a 1D longitudinal plane wave in an infinite, lossless medium, Newton’s second law states that the net force = mass * acceleration.

 

 

 

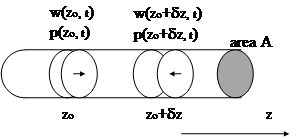
In the above figure, w(z,t) is displacement and p(z,t) is pressure. Therefore,


where r is the density. By taking dz ® 0 and using Bulk modulus (B), we have

By taking Fourier transform of the above equation (with respect to time) we have a general solution of the wave equation (in temporal frequency domain)

where  is the propagation speed of the particle displacement wave. In time domain, we have

In other words, the first term represents a wave traveling to the right and the second to the left.

Since the particle velocity u(z,t) is defined as , in the temporal frequency domain we have

It is then straightforward to see that

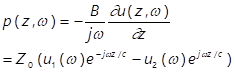
where  and is called the characteristic impedance of the medium. Note the analogy between pressure, particle velocity and voltage, current.

• Reflection and Refraction

 

• A general expression for complex impedance

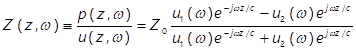
If there is only propagation to the right (or left), then the above equation can be reduced to  ( or ).

• Considering an initial wavefront propagating to the right in the following case,

 

 

 

 

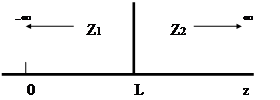
since both pressure and particle velocity are continuous functions and medium 2 is infinite to the right, we have , i.e.,

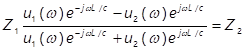
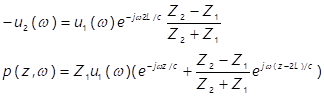
At the boundary (i.e., z=L), we have

Since medium 2 is infinite to the right, the above equation is also the pressure wave in medium 2.

• Now we can define the reflection coefficient and transmission as the following

 

• For two-dimensional cases (i.e., where the incidence angle may not be normal) we have (ignoring shear waves)

 

 

 

 

 

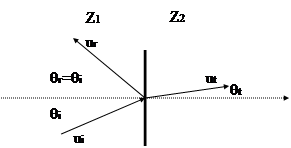
Since the normal components of the particle velocity at the boundary must be continuous, then . Additionally, since the pressure is also continuous at the boundary (i.e., ), it is then straightforward to obtain the following relations

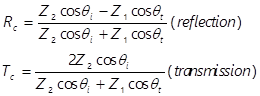
Note that at normal incidence, the above equations reduce to the 1D equations.

• Refraction : As in optics, we can apply Snell’s law

 

where c1 and c2 are the propagation velocities in medium 1 and 2, respectively. If c1 > c2 , a critical angle can be defined as . For any incidence angle greater than the critical angle, total reflection occurs ( i.e., there is no transmission).

 

• Impedance Matching

• It was previously shown that reflection occurs at the boundary between two mediums with different acoustic impedance values. Fortunately, a matching layer can be inserted in between the two mediums in order to avoid reflection at the boundaries. As shown in the following drawing, we would like choose L and Z1 to achieve this goal.

 

 

 

 

In general, impedance in medium 1 (Z(z,w)) is a complex value

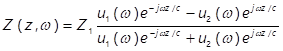
To avoid reflection at boundaries, we need to have

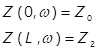
Combining the above equations, we have

where . Note that by choosing 
 for n=0,1,2,…
Zo is real and . Normally, n is chosen to be 0 and this is called “quarter wavelength impedance matching”. Note that if , then and this is a trivial case of no discontinuities. Also note that for multiple layers, impedance values can be found by iterations.

• Two commonly used units

 

            - Pa (Pascal, pressure) :
         - Rayl (acoustic impedance) :